Understanding Fractions

Lesson Objective

Need help in understanding fractions? This lesson will show you the basic ideas on fractions and the common terms used in describing fractions...

About This Lesson

To begin understanding fractions, this lesson introduces you the basic ideas behind fractions.

This lesson will also shows the common terms used in describing fractions. These terms are:

Numerator
Denominator
Proper Fractions
Improper Fractions
Mixed Fractions

You can proceed by reading the study tips first or watch the math video or try out the practice questions.

$proper, improper and mixed fractions$

Study Tips

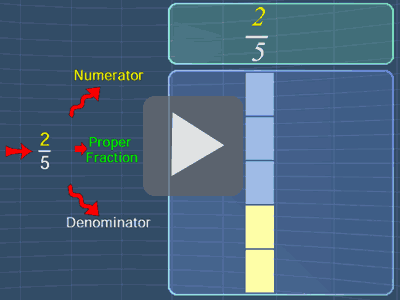
Tip #1 - Numerator and Denominator

When we divide two numbers, we will usually write the expression this way:

2÷5

Now, the above expression can also be written in the form of fraction as shown in the picture on the right.

Notice that, the fraction is divided into 2 parts. The upper part is the numerator and the lower part is the denominator.

The math video below will explain more.

$fraction's numerator and denominator$

Tip #2 - Proper Fractions

When a fraction's numerator is lesser than the denominator, this fraction is a proper fraction. Below are some examples:

⁵/₂₀, ³/₈, ⁷/₈

$proper fraction$

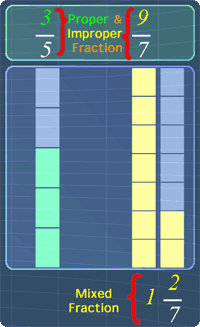
Tip #3 - Improper Fractions & Mixed Fractions

A fraction is considered as an improper fraction when its numerator is greater or equals to the denominator. Below are some examples:

⁸/₇, ²/₁, ²⁰/₆ , ⁵/₅

Note that, mixed fraction is actually equivalent to proper fraction. The only difference is the way they are written.

The picture on the right illustrates this. The math video below will explain more on this.

$Improper and mixed fraction$

Math Video

Lesson video

Math Video Transcript

Understanding Fractions Transcript 

00:00:03.020
This lesson shows you basic ideas behind in understanding fractions.

00:00:07.230
Let's say that we are given the expression, 2 divide 5.   

00:00:12.190
Now, we should know that, 2 divide 5 can be changed into the form of fraction, which is, 2 over 5.

00:00:21.110
In this form, the upper part of the fraction is called, numerator, and the lower part is called, denominator. 

00:00:30.030
Now, since the numerator is smaller than the denominator, this fraction is a proper fraction.

00:00:36.220
Next, let's try to understand what does fraction means.

00:00:42.070
To do so, consider this long piece of bar.

00:00:46.190
When the fraction has a denominator of 5, this means that this bar is divided into, one, two, three, four, five 

small equal parts.

00:00:57.240
When the numerator is 2, it means that we selected 2 out of 5 parts of the bar, shown by the yellow colored parts .

00:01:07.010
Now, when we increase the numerator to three, four, five, observe the number of the yellow parts increase as well. 

00:01:15.240
Here, notice that the numerator, and the denominator, are now the same. 

00:01:21.080
This kind fraction means that, we are dividing 5 with 5, which gives 1.

00:01:28.050
We can visualize this by seeing the entire bar as 1 yellow piece.

00:01:35.050
Let's examine further, when we increase the numerator from 5 to 6, the numerator is now greater than the 

denominator.  

00:01:43.160
Here, we can visualize the fraction, 6 over 5, by seeing this yellow bar as 1, and the next bar has 1 of the 5 parts 

as yellow. 

00:01:54.050
Now, let's further elaborate this fraction.  By now, we should know that 6 over 5 is the same as 6 divides 5.

00:02:04.060
Also, from what we have seen here, 6 over 5 is also the same as one, one over five.

00:02:12.150
The next thing that we should know, since the numerator is greater than the denominator, this fraction is an 

improper fraction.

00:02:20.220
Also, when 6 over 5 is written in this way, this fraction is called a mixed fraction.

00:02:29.110
Alright, let us continue to increase this numerator . Observe how the improper fraction and mixed fraction changes. 

00:02:37.210
The improper fraction, 7 over 5, will have the mixed fraction as 1, 2 over 5.

00:02:45.080
The improper fraction, 8 over 5, will have the mixed fraction as 1, 3 over 5.

00:02:52.110
The improper fraction, 9 over 5, will have the mixed fraction as 1, 4 over 5.

00:03:00.000
The improper fraction, 10 over 5 is the same as 10 divided by 5, which gives 2. 

00:03:07.050
The improper fraction, 11 over 5, will have the mixed fraction as 2, 1 over 5.

00:03:15.060
Alright, that is all for this lesson. Try out the practice question to further your understanding.

--End of Understanding Fractions transcript--

Practice Questions & More

Multiple Choice Questions (MCQ)

Now, let's try some MCQ questions to understand this lesson better.

You can start by going through the series of questions on Understanding Fractions or pick your choice of question below.

Question 1 on the basics terms needed in understanding fractions

Return to Home Page