Solving Linear Equations - Part 2
Lesson Objective
This lesson shows you some examples on how to
solve
linear equations to further your understanding
in this topic.
About This Lesson
This lesson is a continuation from Part 1
for this topic.
After learning the basic concepts behind solving linear equation, it's time to take a look at some examples to understand these concepts better.
After learning the basic concepts behind solving linear equation, it's time to take a look at some examples to understand these concepts better.

Study Tips
Tip #1
Remember that when solving a linear equation,
whatever terms that are added to one side of the
equation, the same terms must be added to the
other side of the equation.
This is important to to keep the equation balanced.
This is important to to keep the equation balanced.
Tip #2
When we multiply both sides of the
equation, it is important to multiply 'all'
the terms in the equation. For example, when
we multiply the following equation with 3:
2x + 1 = 4x -8
The equation becomes:
3(2x +1) = 3(4x -8)
Notice that we put brackets around all the terms for both sides of the equation. By doing so, the equation can remain balanced.
2x + 1 = 4x -8
The equation becomes:
3(2x +1) = 3(4x -8)
Notice that we put brackets around all the terms for both sides of the equation. By doing so, the equation can remain balanced.
Tip #3
Similarly, when we divide both sides of the
equation, it is important to divide all
the terms in the equation. For example, when
we divide the following equation with 3:
2x + 1 = 4x -8
The equation becomes:
(2x +1)/3 = (4x -8)/3
Notice that we need put brackets around all the terms for both sides of the equation. By doing so, the equation can remain balanced.
2x + 1 = 4x -8
The equation becomes:
(2x +1)/3 = (4x -8)/3
Notice that we need put brackets around all the terms for both sides of the equation. By doing so, the equation can remain balanced.
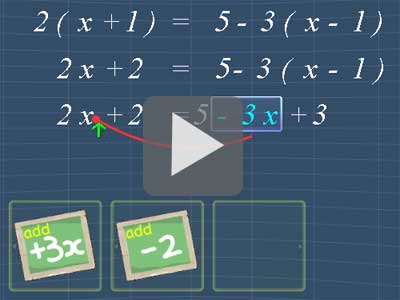
Math Video
Practice Questions & More
Multiple Choice Questions (MCQ)
Now, let's try some MCQ questions to understand
this lesson better.
You can start by going through the series of questions on solving linear equations or pick your choice of question below.
You can start by going through the series of questions on solving linear equations or pick your choice of question below.
- Question 1 on the basics
- Question 2 on the basics