
Solving Linear Equations Practice Question

Question 2
Solve the following linear equation:
Answer
Select and check your answer...
Check Answer
Step by Step Solution
Step 1
It is easier to find x if we remove the
denominator, 3, from the equation. To do
so, we can multiply both sides of the
equation by 3.
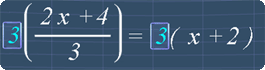
Step 2
Now, both 3 cancel off each other. We
successfully removed the denominator.
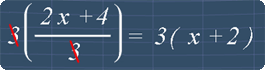
Step 3
The equation becomes:
Notice that, to continue further, we need to
remove the brackets in the Right hand
Side (RHS).
Step 4
Now, 2 multiply by x gives 3x.

Step 5
Next, 3 multiply by +2 gives +6.
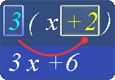
Step 6
The equation becomes:
Step 7
Notice that we can remove 3x by adding
-3x to both sides of the equation. Now
the equation becomes:
2x -3x +4 = 3x -3x +6
On the RHS, 3x and -3x cancel off. We now have:
2x -3x +4 = 3x -3x +6
On the RHS, 3x and -3x cancel off. We now have:
Step 8
We can add 2x with -3x. This gives -x
Step 9
Also, notice that we can remove +4 on
the LHS by adding -4 to both sides of the equation. Now
the equation becomes:
2x -3x +4 -4 = 6 -4
+4 and -4 cancel off. We now have:
2x -3x +4 -4 = 6 -4
+4 and -4 cancel off. We now have:
Step 10
Now, on the RHS, minus 6 with 4 gives 2.
The equation becomes -x = 2.
The equation becomes -x = 2.
Step 11
Now, we can get x by multiplying
both sides by
-1.
The equation becomes:
Step 12
Now, -1 multiplying by -x gives x.
Similarly, -1 multiplying by 2 gives -2.
Therefore we get x = -2. The answer is C.
Similarly, -1 multiplying by 2 gives -2.
Therefore we get x = -2. The answer is C.
To close all the steps, reload the solving linear equations question page