
Volume Formulas
Formula
The lesson on the volume of a cube will
explain the basics needed. Here's a summary:
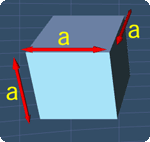
All the sides (edges) of a cube have the same length. If each side has the length a, the formula for the volume V will be:
All the sides (edges) of a cube have the same length. If each side has the length a, the formula for the volume V will be:
Example
Given a cube where the length of each side (edge) is 3cm. Find the volume of this cube.
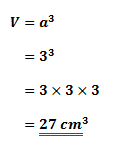
Formula
The lesson on How to Find the Volume
will cover the
basics needed to find the volume of a rectangular solid. Here's a short summary:
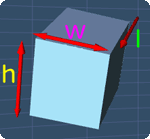
A rectangular solid has the width w, length l and height h. The volume V for this solid is the multiplication of the width, height and length together. This gives:
A rectangular solid has the width w, length l and height h. The volume V for this solid is the multiplication of the width, height and length together. This gives:
Example
Given a rectangular solid with the width 2ft, length 3ft, and height
5ft. Find
its volume.
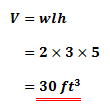
Formula
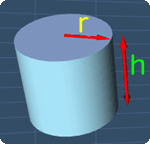
The lesson on the volume of a cylinder
will explain the basic ideas needed to use this formula. Here's a short summary on
it:
For a cylinder that has the radius r and height h, its volume will be:
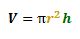
Where π is constant that is approximately equals to 3.14.
For a cylinder that has the radius r and height h, its volume will be:
Where π is constant that is approximately equals to 3.14.
Example
Given a cylinder with the radius 3cm and height 5cm. Find its volume.
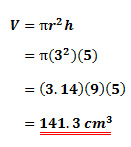 Take π = 3.14.
Take π = 3.14.
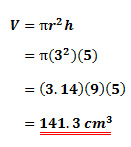
Formula

The lesson of the volume of a sphere will
show the basic ideas on using this formula. Here's a summary:
For a sphere with the radius r, the volume V is given as:
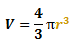
Where π is constant that is approximately equals to 3.14.
For a sphere with the radius r, the volume V is given as:
Where π is constant that is approximately equals to 3.14.
Example
Given a sphere with the radius 3 inches. Find the volume of this sphere.
Take π = 3.14.
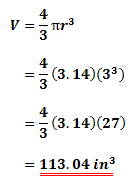
Take π = 3.14.
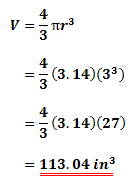
Formula
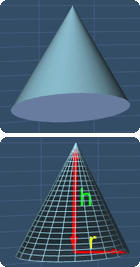
The lesson on the volume of a cone will show you the basics needed to use the
formula. Note that, the volume of a cone is just 1/3 of the volume of a
cylinder.
Consider a cone with the base radius r and height h. The volume V for this solid is simply:
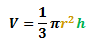
Where π is constant that is approximately equals to 3.14.
Consider a cone with the base radius r and height h. The volume V for this solid is simply:
Where π is constant that is approximately equals to 3.14.
Example
Given a cone with the base radius 2cm and height
3ft. Find
its volume.

Formula
The lesson on the volume of a
pyramid will explain the basic needed to use this formula. Note that, the
volume of a pyramid is actually 1/3 of the volume of a rectangular solid.
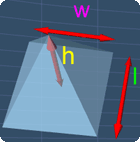
Now, for a pyramid with the width w, length l and height h, the volume, V of the pyramid will be:
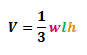
Now, for a pyramid with the width w, length l and height h, the volume, V of the pyramid will be:
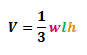
Example
Given a pyramid with the width 3ft, length 4ft and height 5ft. Find its volume.
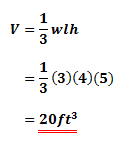
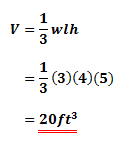
Return to Home Page from
Volume Formulas