Khan Academy on a Stick : Limits

Khan Academy on a Stick

Calculus

Introduction to limits
cc

Introduction to limits
Limit at a point of discontinuity
cc
Determining which limit statements are true
cc
Limit properties
cc
Limit example 1
cc
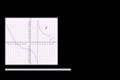One-sided limits from graphs

Limits

Limits are the core tool that we build upon for calculus. Many times, a function can be undefined at a point, but we can think about what the function "approaches" as it gets closer and closer to that point (this is the "limit"). Other times, the function may be defined at a point, but it may approach a different limit. There are many, many times where the function value is the same as the limit at a point. Either way, this is a powerful tool as we start thinking about slope of a tangent line to a curve. If you have a decent background in algebra (graphing and functions in particular), you'll hopefully enjoy this tutorial!

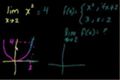Introduction to Limits
cc

Introduction to the intuition behind limits
Limit Examples (part 1)
cc

Some limit exercises
Limit Examples (part 2)
cc

More limit examples
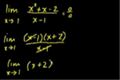Limit Examples (part 3)
cc

More limit examples
Limit Examples w/ brain malfunction on first prob (part 4)
cc

3 interesting limit examples (correct answer for problem 1 is 3/16 (6/(4*8) NOT 6/(4+8))
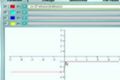More Limits
cc

More limit examples

Old limits tutorial

This tutorial covers much of the same material as the "Limits" tutorial, but does it with Sal's original "old school" videos. The sound, resolution or handwriting isn't as good, but some people find them more charming.

Limits and infinity

You have a basic understanding of what a limit is. Now, in this tutorial, we can explore situation where we take the limit as x approaches negative or positive infinity (and situations where the limit itself could be unbounded).

Squeeze Theorem
cc

Intuition (but not a proof) of the Squeeze Theorem.
Proof: lim (sin x)/x
cc

Using the squeeze theorem to prove that the limit as x approaches 0 of (sin x)/x =1

Squeeze theorem

If a function is always smaller than one function and always greater than another (i.e. it is always between them), then if the upper and lower function converge to a limit at a point, then so does the one in between. Not only is this useful for proving certain tricky limits (we use it to prove lim (x → 0) of (sin x)/x, but it is a useful metaphor to use in life (seriously). :) This tutorial is useful but optional. It is covered in most calculus courses, but it is not necessary to progress on to the "Introduction to derivatives" tutorial.

Limit intuition review
cc
Building the idea of epsilon-delta definition
cc
Epsilon-delta definition of limits
Proving a limit using epsilon-delta definition
Limits to define continuity
cc
Epsilon Delta Limit Definition 1
cc

Introduction to the Epsilon Delta Definition of a Limit.
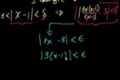Epsilon Delta Limit Definition 2
cc

Using the epsilon delta definition to prove a limit

Epsilon delta definition of limits

This tutorial introduces a "formal" definition of limits. So put on your ball gown and/or tuxedo to party with Mr. Epsilon Delta (no, this is not referring to a fraternity). This tends to be covered early in a traditional calculus class (right after basic limits), but we have mixed feelings about that. It is cool and rigorous, but also very "mathy" (as most rigorous things are). Don't fret if you have trouble with it the first time. If you have a basic conceptual understanding of what limits are (from the "Limits" tutorial), you're ready to start thinking about taking derivatives.